Dr Markus Schmuck
resume

PhD Mathematics, Universität Tübingen, Germany (advisor: Andreas Prohl); MS, Mathematics, ETH Zurich, Switzerland. Now holds a faculty position in Mathematics at Heriot-Watt University in t he School of Mathematical and Computer Sciences
About
Following Imperial position Markus joined as Assistant Professor the faculty at the Department of Mathematics, Heriot-Watt University, Edinburgh, UK. Now Analysis, Research, and Development in Science and Engineering at Federal Administration, Duebendorf, Switzerland. The information below was of relevance whilst a postdoctoral research associate in the Complex Multiscale System group.
I was a research associate of Professor Serafim Kalliadasis and Dr Gregorios A. Pavliotis in the Departments of Chemical Engineering and Mathematics at Imperial College London. Prior to this, I was a postdoctoral fellow with Professor Martin Bazant at MIT with a prospective researcher grant from the Swiss National Science Foundation (SNSF). I obtained my PhD under the supervision Professor Andreas Prohl at the University of Tübingen.
My expertise is in the broad area of partial differential equations including the well-posedness, characterization of solutions with different regularity properties, understanding and elucidating their underlying physical principles, and finally their effective and reliable computation (discretization). In my research, I apply rigorous mathematical tools to justify and physically derive of new models, upscaled, homogenized and effective macroscopic equations. The herewith gained analytical and physical experience allows to systematically and reliably propose computational strategies based on finite elements for instance.
Email address: m.schmuck@imperial.ac.uk
Current Research
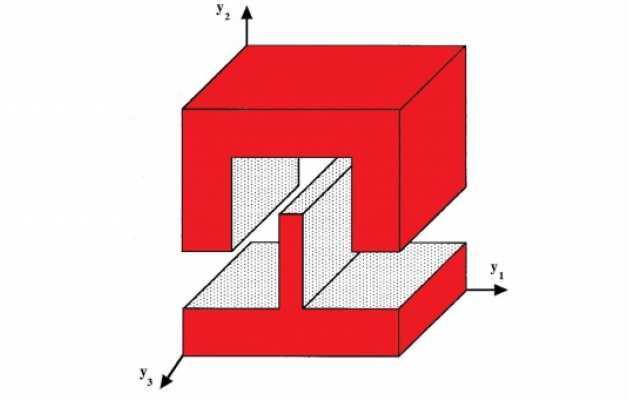
Multiscale analysis and modeling: Derivation of effective, reliable, and low dimensional upscaled equations for problems described by ordinary, partial, and stochastic partial differential equations.
Examples: Upscaled/homogenized phase field equations for porous and heterogeneous media and microfluidic devices; stochastic mode reduction of the generalized Kuramoto-Shivasinsky equation.
Exploit scale effects for the search for new physics which can lead to promising new directions for science, engineering, and industrial purposes.
Examples: Emergence of a new transport term in the porous media approximation of the Nernst-Planck-Poisson system by exploiting the strong heterogeneity in material properties.
Apply thermodynamic (free energies, laws of thermodynamics), physical, and mathematical principles (gradient flows, optimal transport) to base ad hoc and phenomenologically accepted principles on a proper rigorous foundation.
Example: Complex multiphase systems such as immiscible fluids with suspended particles.
Below there are some images from my research: