Research Team
- Professor Ahmer Wadee: Imperial College London
- Professor Leroy Gardner: Imperial College London
- Dr Li Bai: Former Research Student
- Dr Maryam Farsi: Former Research Student
- Elizabeth Liu: Research Student
- Jiajia Shen: Research Student
Background
A series of analytical models that describe the behaviour of a variety of structural components and systems, which are known to suffer from interactive buckling, have been studied. The aim of the work is to use mathematical modelling for interpreting the mechanical response of highly nonlinear problems. Results from the nonlinear structural analysis of perfect systems that exhibit complex instability phenomena can be obscured by standard numerical modelling such as the finite element method. Typical features including symmetry breaking, the localization of post-buckling modes and progressive cellular buckling [1], sometimes known as "snaking"; an important topic in nonlinear systems which pervades many branches of applied mathematics, structural mechanics and physics (especially nonlinear optics).

Recently, the interaction of lateral-torsional buckling (LTB) and local buckling has been investigated through the development of an analytical model that has been validated through experimental studies, the work being published in the Proceedings of the Royal Society A [2]. Unlike the work on sandwich structures[3] the localization of the buckling patterns is only the beginning of the story with the equilibrium response showing, sequentially, an initial critical bifurcation, a secondary bifurcation and then a series of unstable "snap-backs" that represent the appearance of a new buckling cell appearing. A similar finding has been published for I-section columns [4] and stiffened plates [5] both under pure compression.
Context and Methodology
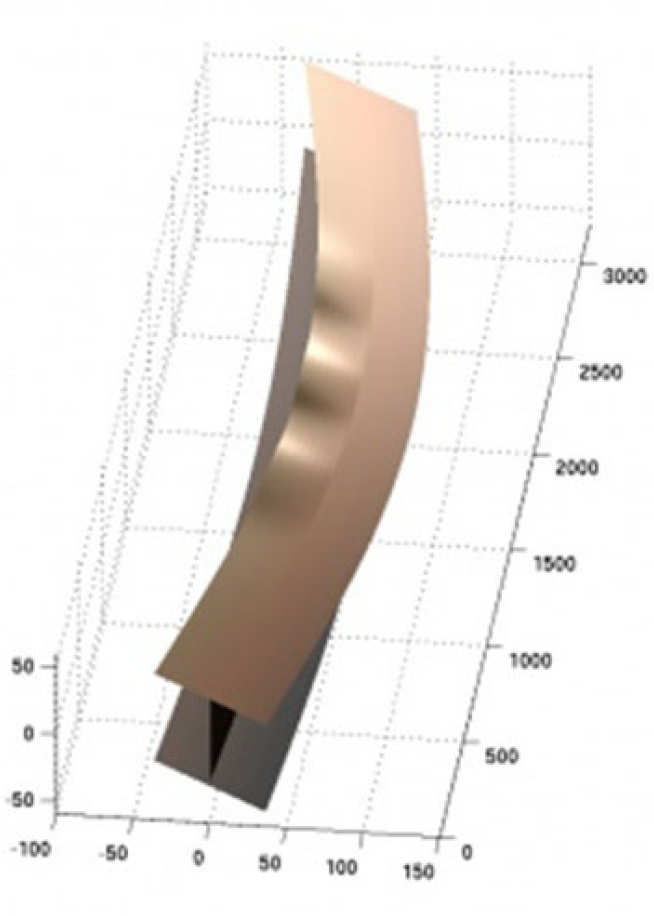
The governing equilibrium equations can be derived from variational principles by minimizing the total potential energy from continuous functional variables and modal descriptions. The resulting system of differential and integral equations are solved numerically using the excellent continuation package AUTO. The model describing the interaction between LTB and local buckling has been found to agree with a series of newly conducted experiments and a series of classic experiments published by Cherry [6] in 1960. The model describing the interaction between local and Euler buckling has been compared to experiments performed by Jurgen Becque and Kim Rasmussen [7] at the University of Sydney; the work being published in 2009.
Short movie of progressive LTB and local buckling deformation (model simulation)
Seminars and Conference Presentations
- July 2015
Lisbon, Portugal. 8th International Conference on Advances in Steel Structures (ICASS 2015).
Two talks: one delivered by Elizabeth Liu and one delivered by Jiajia Shen.
Madrid, Spain. 9th European Solid Mechanics Conference (ESMC 2015). - September 2014
Cardiff, UK. Institute of Physics Workshop on Lightweight Structures. - August 2014
Hamilton, Ontario, Canada. ASCE Engineering Mechanics Conference (EMI 2014). - September 2013
Cape Town, South Africa. 5th International Conference on Structural Engineering, Mechanics and Computation (SEMC 2013). - August 2013
Evanston, Illinois, USA. ASCE Engineering Mechanics Institute Conference (EMI 2013). - December 2012
Glasgow, UK. 6th International Conference on Coupled Instabilities in Metal Structures (CIMS 2012). Two talks: one delivered by Ahmer Wadee and one delivered by Li Bai. - September 2012
Dubrovnik, Croatia. 11th International Conference on Computational Structures Technology (CST 2012 - Invited) - July 2012
Graz, Austria. 8th European Solid Mechanics Conference (Euromech: ESMC 2012 - Invited) - July 2011
Rome, Italy. 7th European Nonlinear Dynamics Conference (Euromech: ENOC 2011) - June 2011
TU Berlin, Institute of Mechanics. Research Seminar. - March 2011
University of Bath, Centre for Nonlinear Mechanics. Brooke Session.
References
- Hunt, G. W., Peletier, M. A., Champneys, A. R., Woods, P. D., Wadee, M. A., Budd, C. J. and Lord, G. J., 2000. Cellular buckling in long structures. Nonlinear Dyn. 21(1):3-29.
- Wadee, M. A. and Gardner, L., 2012. Cellular buckling from mode interaction in I-beams under uniform bending, Proc. R. Soc. A. 468(2137):245-268.
- Hunt, G. W. and Wadee, M. A. 1998. Localization and mode interaction in sandwich structures. Proc. R. Soc. A. 454:1197-1216.
- Wadee, M. A. and Bai, L., 2014. Cellular buckling in I-section struts. Thin-Walled Struct. 81, 89-100.
- Wadee, M. A. and Farsi, M. 2014. Cellular buckling in stiffened plates. Proc. R. Soc. A. 470, 20140094.
- Cherry, S., 1960. The stability of beams with buckled compression flanges. Struct. Eng. 38(9), 277-285.
- Becque, J. and Rasmussen, K. J. R., 2009. Experimental investigation of the interaction of local and overall buckling of stainless steel I-columns. ASCE J Struct Eng. 135(11):1340–1348.
Contact Structures
Structures
Civil and Environmental Engineering
Skempton Building
Imperial College London
South Kensington Campus
London, SW7 2AZ
Telephone:
+44(0) 207 594 6040
Email: r.bello@imperial.ac.uk
Alternatively view our people lists
We are located in the Skempton Building (building number 27 on the South Kensington Campus Map). How to find us